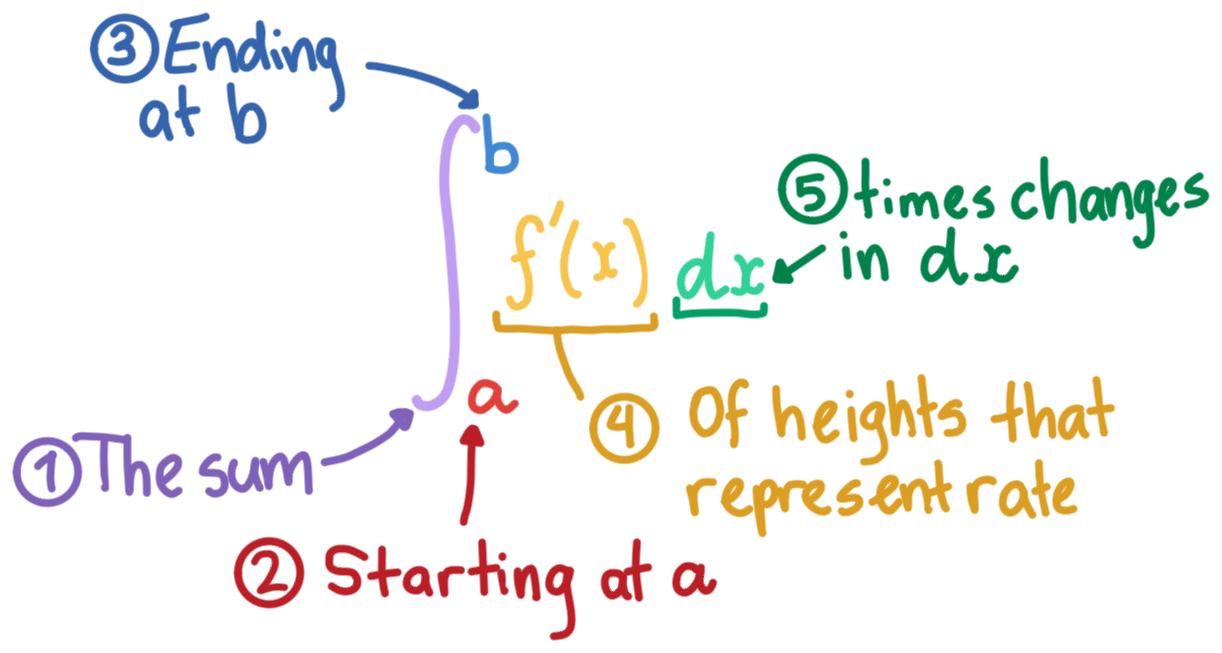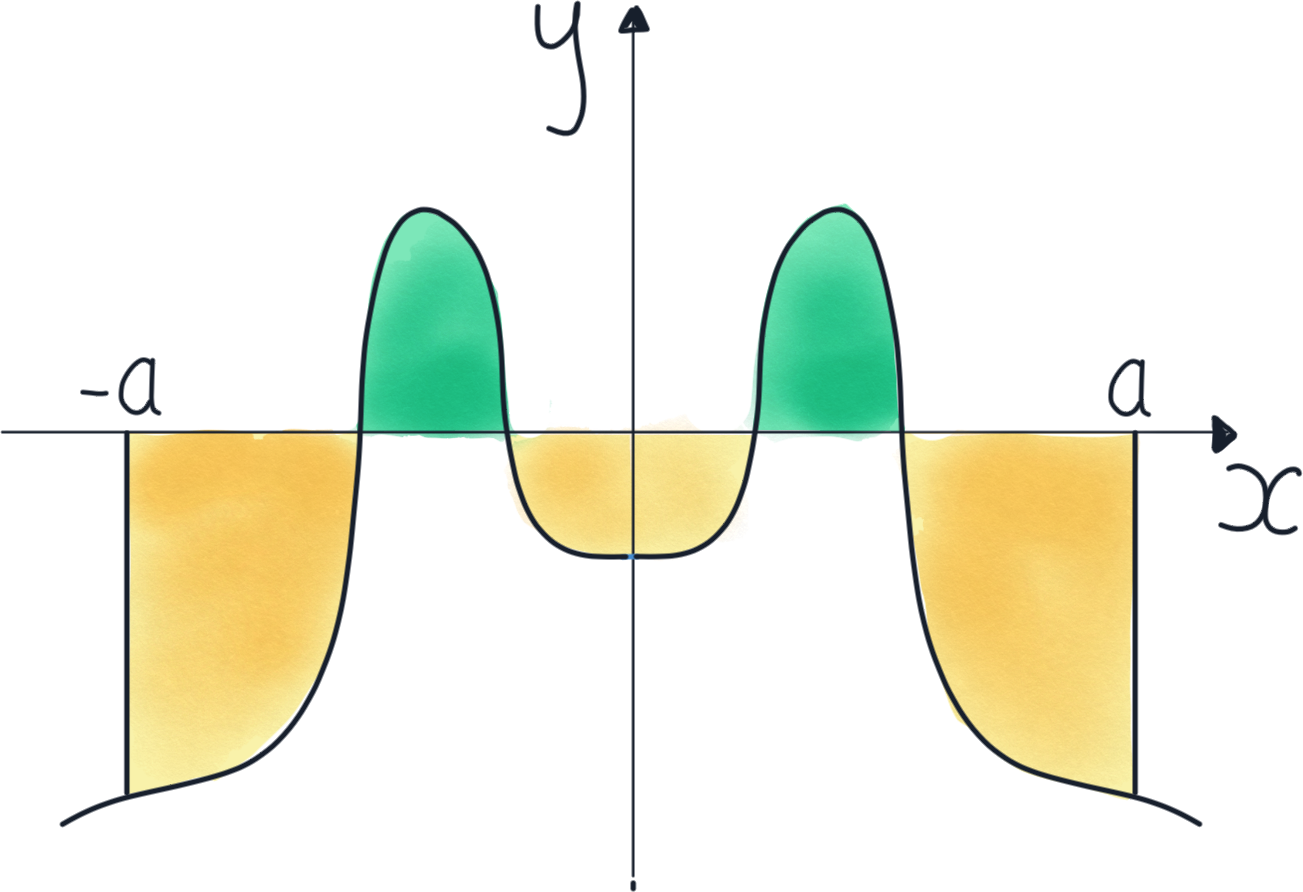Content
Content
This lesson introduces basic rules of integration. By mastering the content in this session, you will be able to:
| |
|
Syllabus Outcomes
C4.2: Areas and the definite integral
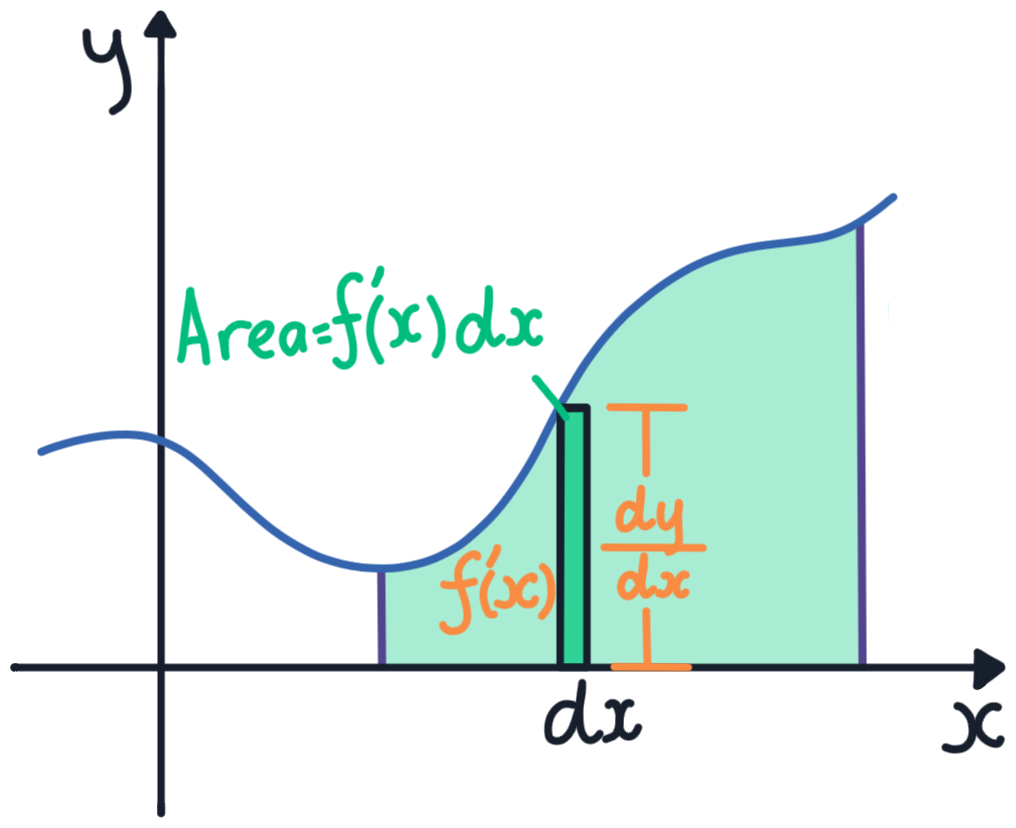
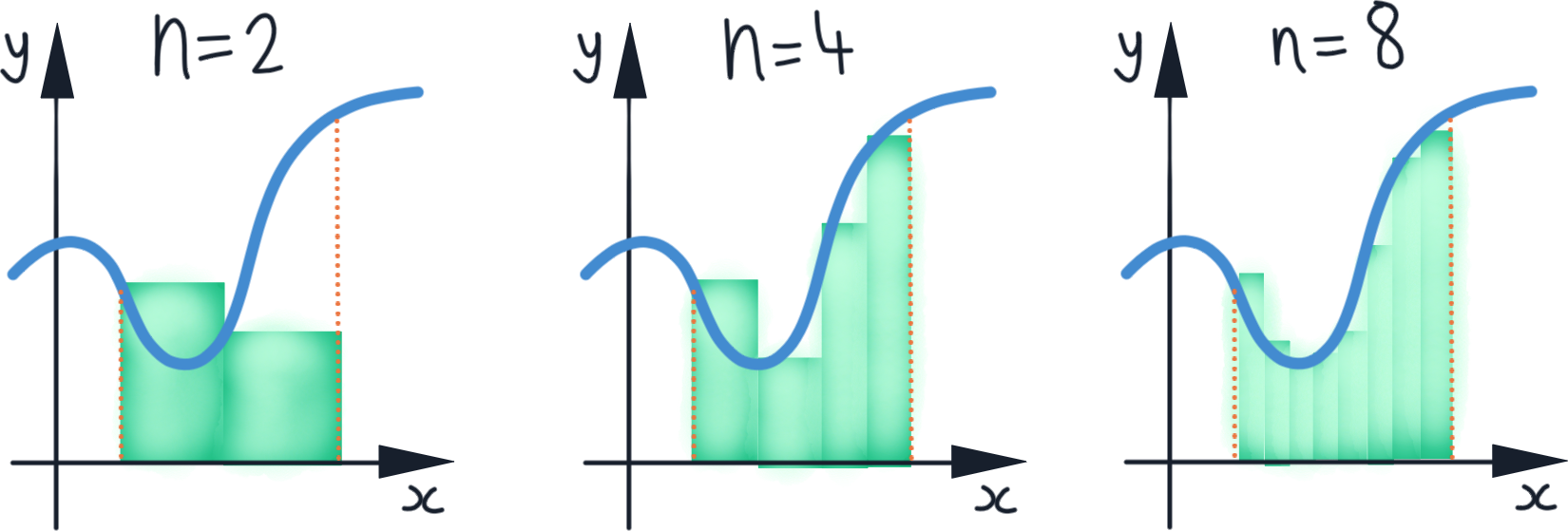
Know that ‘the area under a curve’ refers to the area between a function and the
-axis, bounded by two values of the independent variable and interpret the area under a curve in a variety of contexts; Use the notation of the definite integral
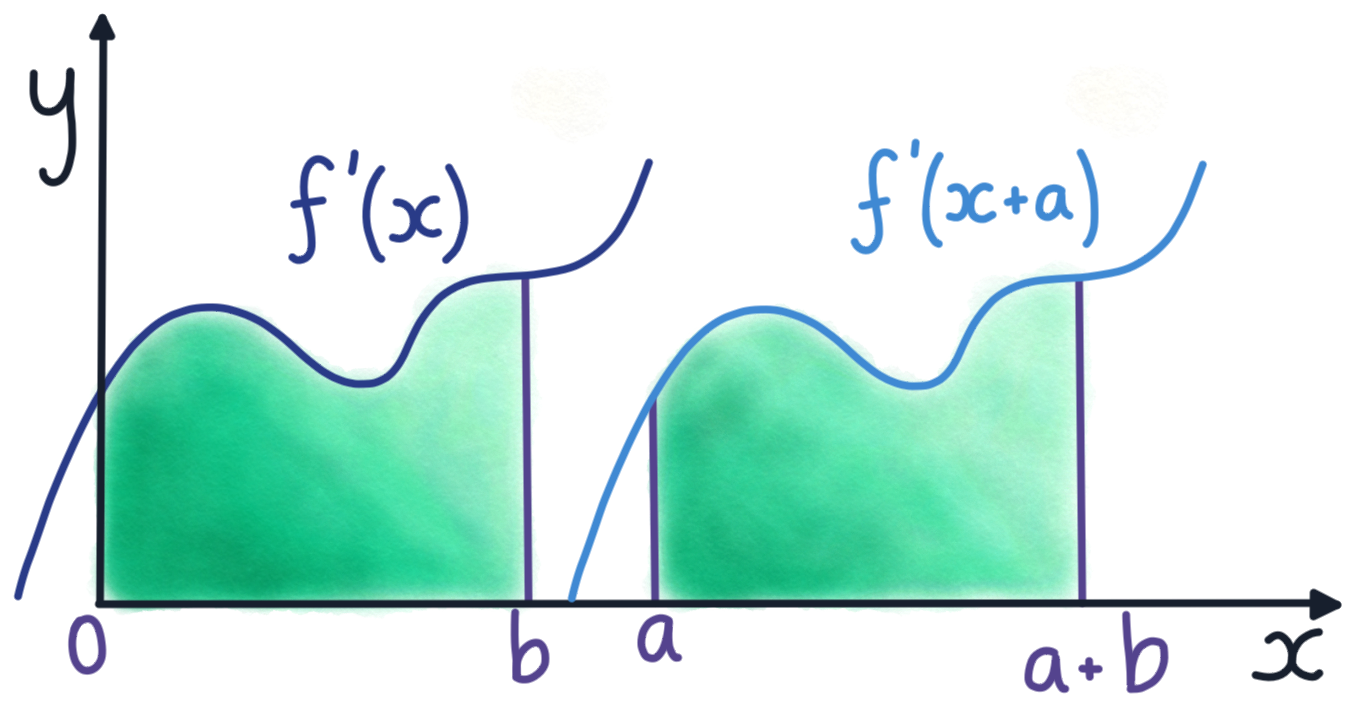
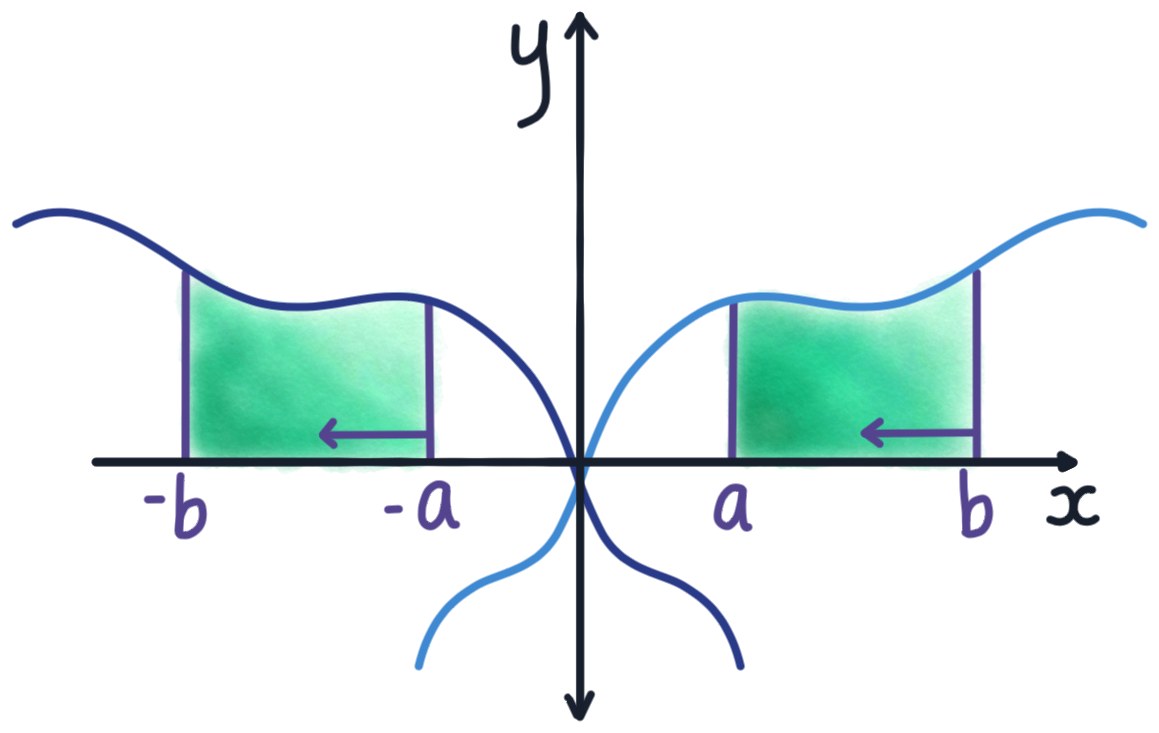
for the area under the curve from to if
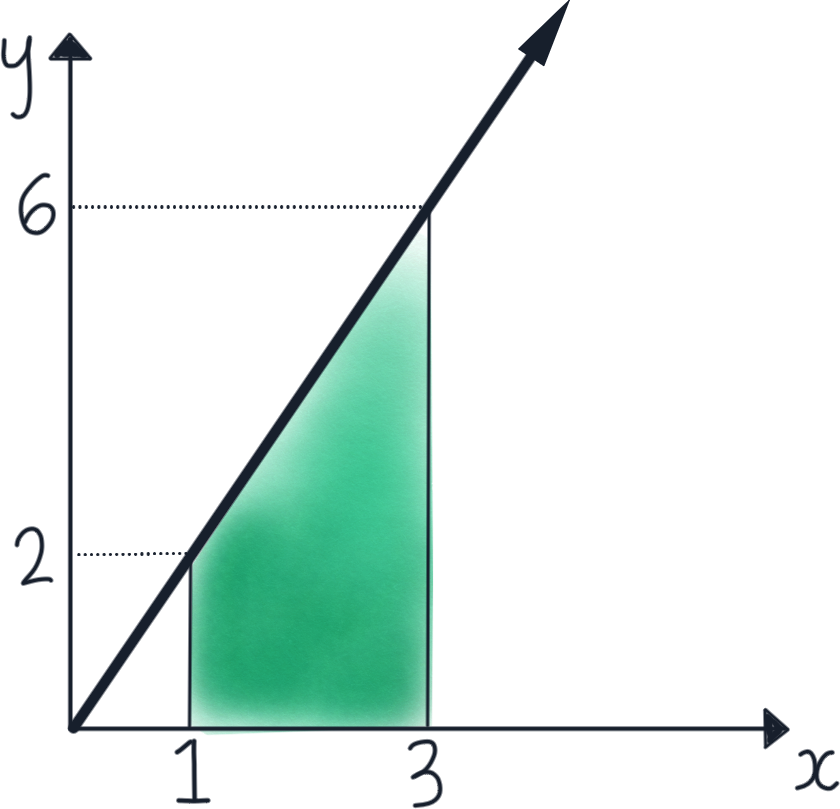
Use geometric ideas to find the definite integral ∫ 𝑓 𝑏 𝑎 (𝑥) 𝑑𝑥 where 𝑓(𝑥) is positive throughout an interval 𝑎 ≤ 𝑥 ≤ 𝑏 and the shape of 𝑓(𝑥) allows such calculations, for example when 𝑓(𝑥) is a straight line in the interval or 𝑓(𝑥) is a semicircle in the interval.
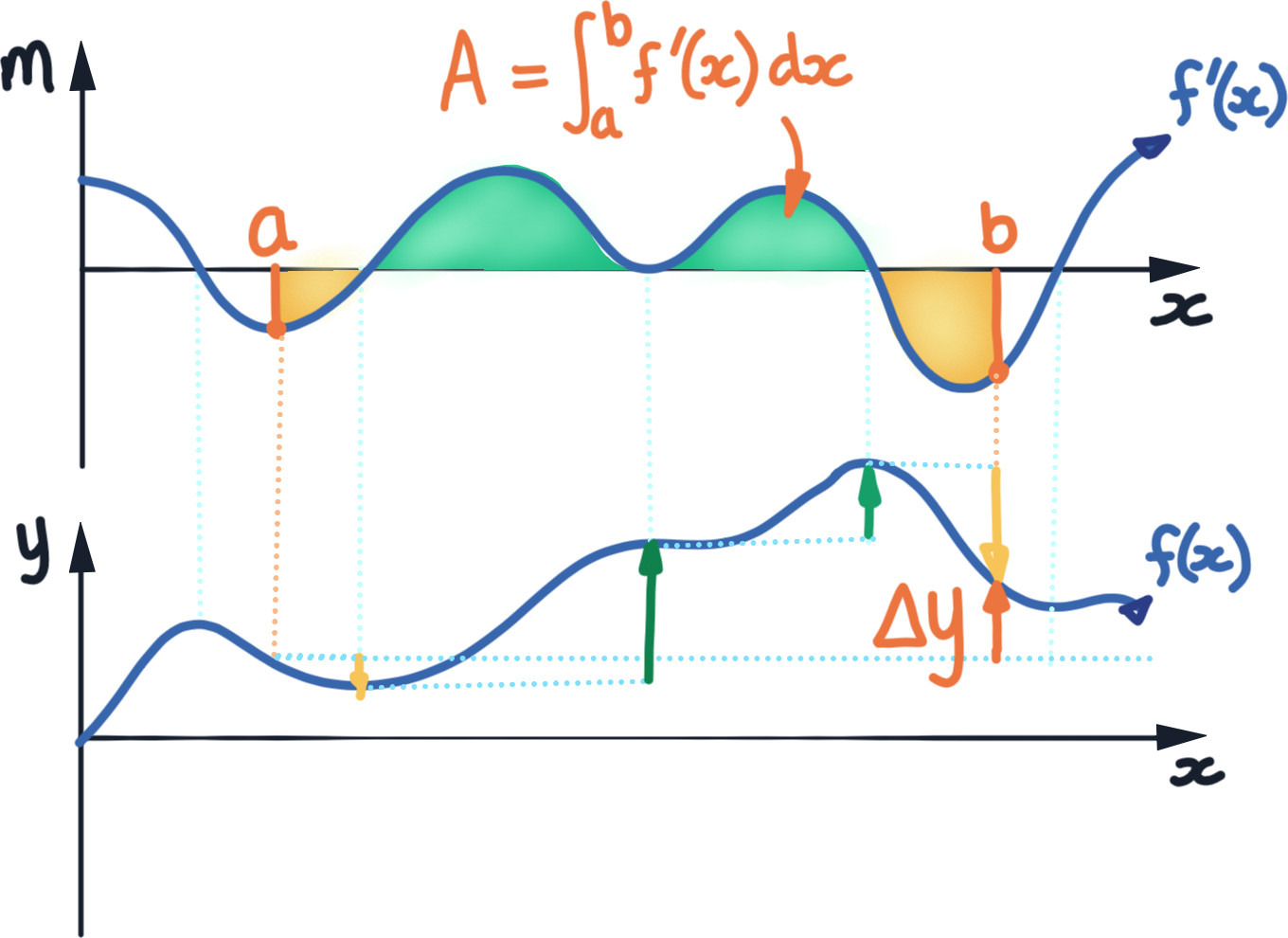
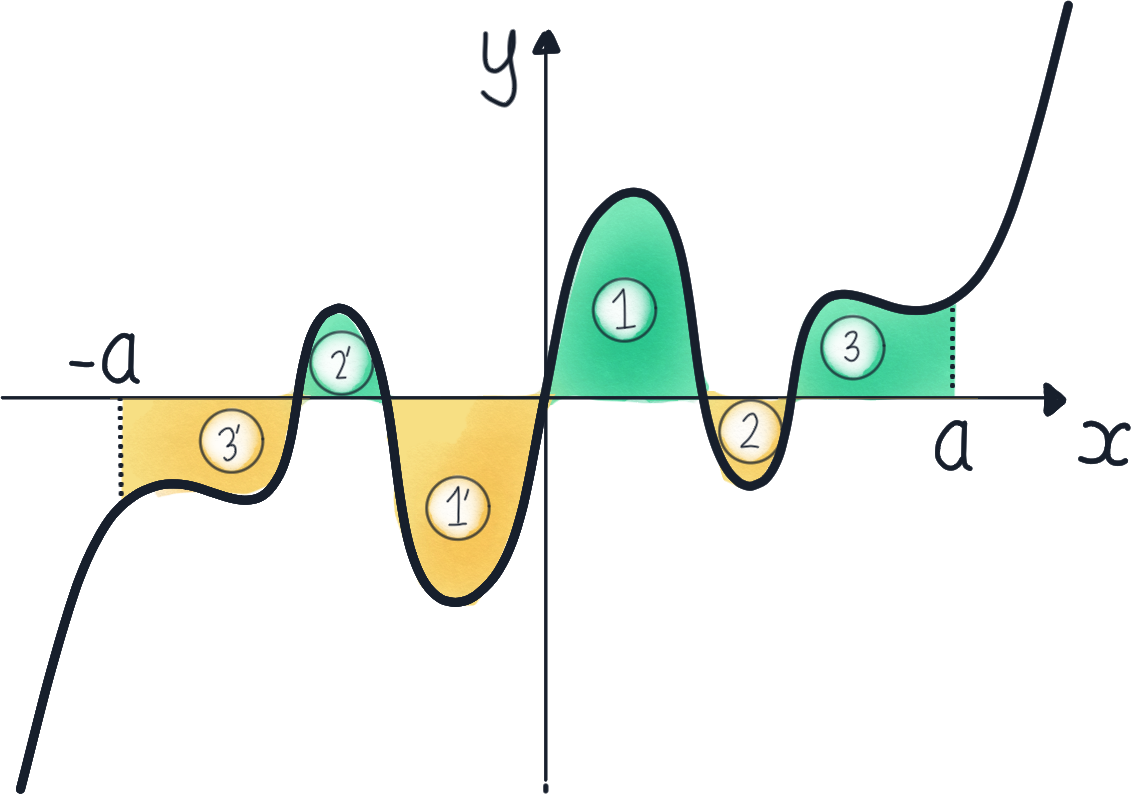
Understand the relationship of position to signed areas, namely that the signed area above the horizontal axis is positive and the signed area below the horizontal axis is negative.
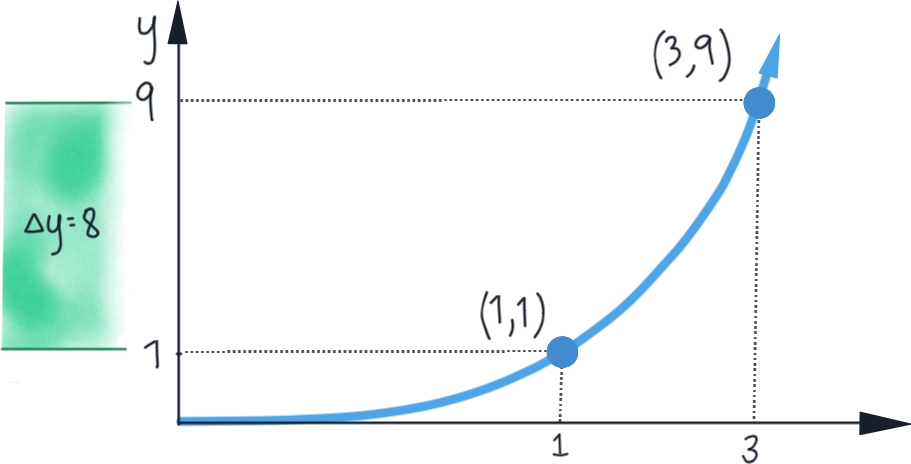
Using technology or otherwise, investigate the link between the anti-derivative and the area under a curve – interpret
as a sum of signed areas. Calculate the area under a curve.
Student Progress
| Name | Prework | Homework |
|---|---|---|